-
Notifications
You must be signed in to change notification settings - Fork 8
New issue
Have a question about this project? # for a free GitHub account to open an issue and contact its maintainers and the community.
By clicking “#”, you agree to our terms of service and privacy statement. We’ll occasionally send you account related emails.
Already on GitHub? # to your account
feasible point solving has a logic error w.r.t. properties of smooth-max #1
Comments
|
Plan for fixing this problem: alpha starts at 1.0 - minimization is done using this value. If the resulting minimum point is not < 0, then double alpha, and try again, etc, until some halting condition. Either alpha exceeds some threshold, or perhaps if two consecutive minimums differ by < epsilon. That is the default logic for alpha. However, it may be the case that the circular constraint Hessian can underflow - the current logic for detecting and preventing underflow can override the value for alpha (set it lower). If this happens, then alpha begins doubling from this corrected value next iteration |
|
Tangentially I think the logic around the circular constraint can be simplified a little, by just declaring radius (r) to be something like 2x(maximum positive value over all constraint functions), as this is likely to overlap at least one constraint surface. In this scheme, scaling the function can be done to make its minimum some standard value, like 1. |
Attempting to add some new constraints for erikerlandson/snowball#1 has turned up a bug in my feasible point algorithm. Specifically, the problem is that I designed this algorithm assuming that smooth-max preserved the location of minima over convex functions. That is to say, if you find the minimum of the maximum over some convex functions, and then find the minimum of the smooth-max over those functions, those two minima will have the same location. This isn't true, as this example with simple 1D functions shows (the green smooth-max curve has its minimum to the right of the corresponding minimum over the true max):
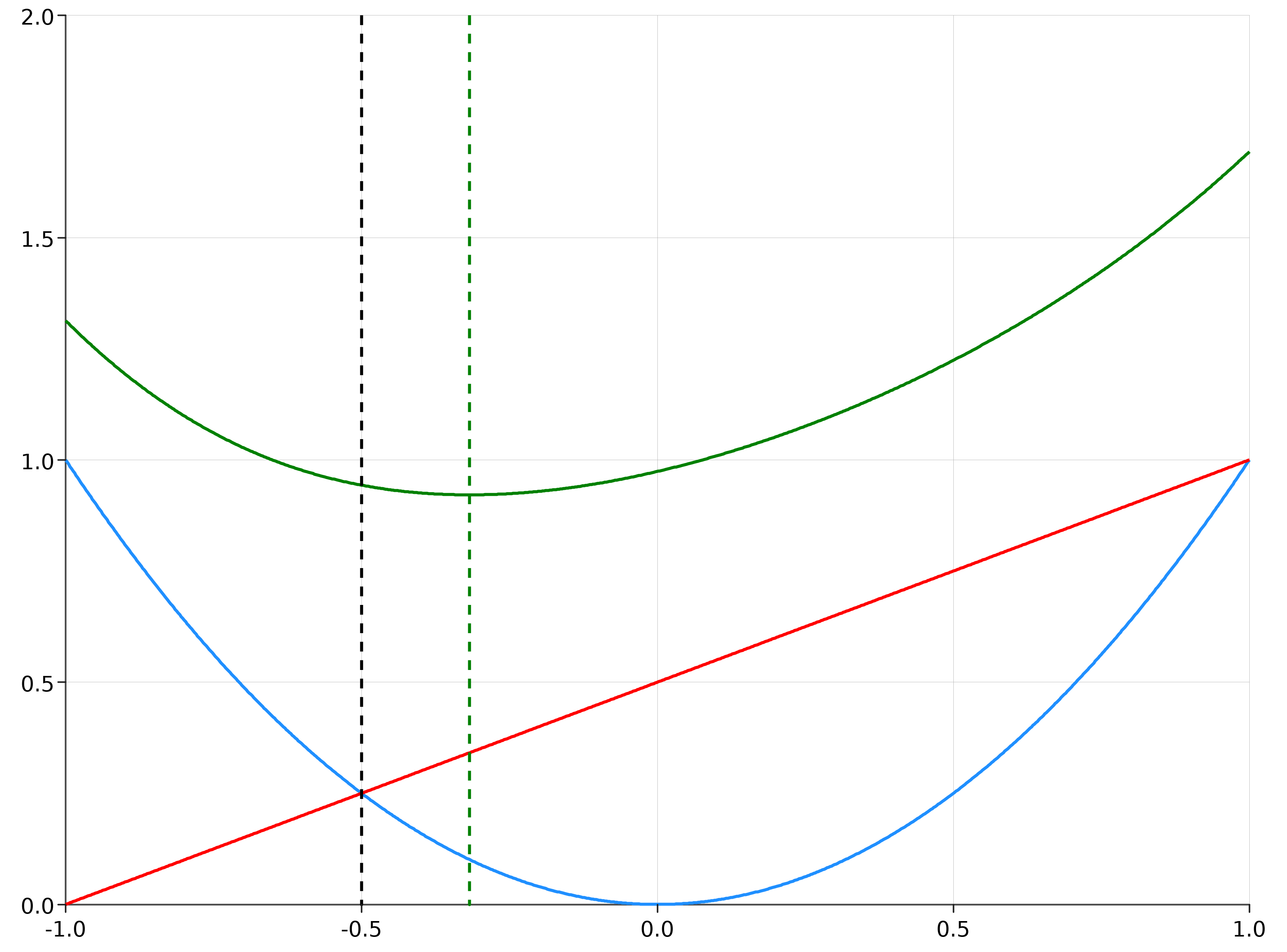
Under some circumstances, this discrepancy will cause the current algorithm to find its minimum outside the actual feasible region and so it will report failure on constraints that actually have a non-empty feasible region.
I have to re-think the way that the
alphaparameter for smooth-max is currently being used, and update the feasible point algorithm.The text was updated successfully, but these errors were encountered: